ACADEMIC ACHIEVEMENTS
Stevan Pilipović

Mihailo Petrović Alas (Elementary School “Mihailo Petrović Alas”)
HAVING GRADUATED FROM THE GRAND School in Belgrade, Mihailo Petrović continued studies of mathematics and physics in Paris, acquiring the degree of “licence” for mathematics and physics. He was the first foreign doctoral student in mathematics at the L’Ecole Normale Supérieure which, at the time and now, is the most famous educational center for mathematics in the world. Petrović’s professors there were the most illustrious mathematicians of that time, including Henri Poincaré, Jean Gaston Darboux, Paul Émile Appel, Paul Tannery, Charles Hermite and Paul Painlevé. In such an environment, the main requirements for success in mathematics were talent, originality of ideas and persistence. Young Mihailo Petrović had all three of these qualities, and this was confirmed by the recognition he received as the best student of his generation. He defended his PhD dissertation in 1894, and his co-supervisors were Charles Hermite and Charles Émile Picard. To show his appreciation, Petrović dedicated his dissertation to Tannery and Painlevé. He thus entered the world of great mathematicians of his time. The Serbian translation of Petrović’s dissertation has been made by the Serbian Academy of Arts and Sciences member Bogoljub Stanković.
Some of the most important results Petrović obtained were already in his doctoral dissertation, therefore we will describe in short the topic of this dissertation and its main result. In his thesis, Petrović studied a class of non-linear first order differential equations, as well as a class of non-linear second order differential equations; the latter are well-known as Painlevé equations. This topic was very popular at the time. In the late 19th century, the most well-known names in French and world mathematics, Picard, Painlevé and Fuchs, studied non-linear second-order equations with no movable branch points. In that context, Painlevé, later Fuchs, and even later (1910) Gambier described the sub-class of these equations which take the following form:
$y”=F(x,y,y’)$
whereby $F$ is the quotient of two polynomials with respect to $y$ and $y’$ with holomorphic functions as coefficients. Painlevé has found fifty general forms which have immovable branch points which he, with the assistance of Fuchs and Gambier, reduced to six essentially new equations. These are the equations that cannot be solved in terms of familiar special functions such as elliptic functions, those that cannot be solved in terms of linear equations and that also cannot be transformed from one into the other. These six equations bear the name Painlevé transcendents. These six generic cases today have a great significance in many areas of analysis, algebra and geometry, but also in applications such as statistical mechanics, physics of plasmas, non-linear wave theory, quantum gravity theory, quantum field theory, theory of relativity and nonlinear optics. Painlevé obtained his first results in papers published in 1887 and 1895. This problem had also been addressed by the other greatest mathematicians of that time, Poincaré and Picard. We particularly emphasize this fact, considering that Mihailo Petrović had defended his doctoral disseration in front of a commission consisting precisely of Picard and Painlevé.


The front cover and the first page of Petrović’s doctoral thesis (University Library “Svetozar Marković”)
The doctoral dissertation of Mihailo Petrović is dedicated to the immovability of zeros, poles and essential singularities in solutions of algebraic first– and second-order differential equations. In accordance with the definition, singularities of solution consist of their poles, essential singularities, final and logarithmic branch points, while movability is defined as a property according to which zeros and singularities are constantly changing as initial conditions of a given equations change. In other words, the property of movability determines whether a problem behaves “well,” unlike the problem which behaves “badly” and which often does not have a solution if initial conditions change. Mihailo Petrović studied problems for which solutions of equations often have singularities independent of the change of initial conditions.
In this first part of his doctoral dissertation, Mihailo Petrović analyzes first-order equations in which one has products of powers of an unknown function y, powers of its derivative $y’$, and of holomorphic functions with respect to the independent variable $x$:
\begin{eqnarray*}
F(x,y,y’) \equiv \sum_{i=1}^{s} \phi_{i}(x)y^{m_{i}}(y’)^{n_{i}}=0.
\end{eqnarray*}
In the statement of the main result of his thesis, an important role is played by a polygon $P$ which Petrović constructs in the following way. Polygon $P$ has an $s$ vertices $(M_{i},N_{i})$, $N_{i} =n_{i}$, $M_{i} =m_{i} + n_{i}$ , $i= 1, \ldots , s$, which have been done according to certain rules, while it designates with $(M_{\alpha},N_{\alpha})$, that is, $(M_{\beta},N_{\beta})$, the most distant i.e. the most proximate vertices to the axis ΟΝ, while λ designates coefficients of the direction of the sides of the polygon. His results subsequently is: Poles and essential singularities of the general solution of the first-order equation $F(x,y,y’)=0$ do not change with the constant of integration if and only if the adjacent polygon does not have any vertex right of the highest elevated vertex of the polygon. For the existence of a movable zero of an $\lambda$ order, it is necessarz and sufficient for the polygon to have a side with an $\lambda$ inclination and to have the moveable pole of an $\lambda$ order, if and only if the polygon has a side with an inclination $-\lambda$.
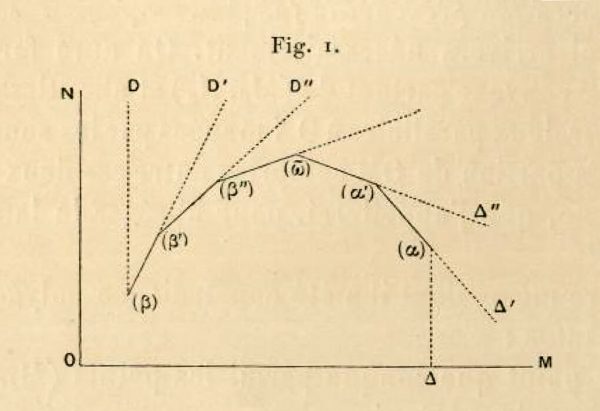
Polygon R, a drawing from Petrović’s dissertation (University Library “Svetozar Marković”)
According to the data analyzed by the historian Dr. Dragan Trifunović, Petrović published 393 papers of which 328 are mathematical manuscripts from twelve different areas in the most prominent journals of that time, as well as today. In academic journal Comtes Rendus, in which, in the opinion of French mathematicians, the very best papers appear, Mihailo Petrović published as much as thirty papers. He has also published his work in Acta mathematica, Mathematische Annalen (two papers), Bulletin de la Sociètè mathèematique de France (fourteen papers), American Journal of Mathematics (three papers), as well as in numerous other Swiss, German, Czech and Polish journals. In terms of the number of published manuscripts in leading international journals, Petrović is still one of the most productive Serbian mathematicians of all times.
Petrović’s first paper anticipated the topic of his doctoral dissertation and the papers which followed subsequently build up on the results from his dissertation. Independence with respect to constants, of singularities, zeros, extremes and some other properties of a general solution dominates in all these papers as the clear determinant of Petrović’s academic work. This property is the essential, constitutive characteristic of the model he is studying, that is, the equation that describes this model. When he describes the residuum of function, studies the so-called binomial equations of the first order, the asymptotics of the solution, writes about “one class of second-order differential equations” or the nature of the solution, to paraphrase titles of some of his papers, internal structural relation between the dependent and the independent variable depicted by the very equation remains the central goal of his explorations. A great creative potential in academic work, displayed in his doctoral dissertation, is also visible in papers written and published in the period of the First World War when Picard, Appel and Hadamard were publishing them in Comtes Rendus. During the First World War, he resided in Switzerland, where he worked as a cryptographer of the Serbian Army Command. Despite this engagement, he has had sufficient energy to also delve in serious mathematical problems.
A quantitative analysis of solutions of differential equations, without solving the equation itself, as well as solving certain classes of equations, represent a significant part of the mathematical opus of Mihailo Petrović. In time, as he started to spend more and more time in Serbia and less and less time at the Sorbonne, we can notice that Petrović was engrafting new ideas on the ideas from his doctoral dissertation, as well as on his extraordinary knowledge of the theory of analytical functions. In most of his initial papers from the late 19th and early 20th century, he mostly elaborated the ideas he has developed during his residence and his scholarly work in France. Naturally, the advantages of residing in France had been great and numerous. There, Petrović had access to the latest and crucial literature, and in addition he had immediate contacts with the greatest mathematicians of that time, with whom he could exchange his ideas and results and discuss them. Considering that in his thesis he particularly studied solutions of the equation $y’= R(t,y)$ with the Painlevé property, he explored in particular various forms of Riccati’s equation
$z'(t) = 2α(t)z(t) + b(t) -c(t)z^2(t),$
which can be solved with integrations and familiar special functions. Fuchs has shown that first-order equations, which were also studied by Petrović and solutions of which contain immovable branch points, boil down to matrix form of the Ricotta equation. Several dissertations by his doctoral students used this fact as a starting point. Petrović’s mathematical achievements are not exhausted with and do not rely exclusively on aforementioned ideas and methods. To him, but also to his doctoral students, comparison theorems for solutions of equations with respect to comparison of coefficients or right-hand side of equations, the so-called Sturm type theorems frequently provided an inspiration. His research often features simple but fine mathematical witticism with which he managed to obtain results of a general character for various classes of linear and nonlinear equations. Asymptotics of the solution, mostly of nonlinear Riccati-type equations, has also occupied an important place in his analysis of equations.
Petrović’s papers belong primarily to the following areas of analysis: differential equations, complex and real analysis. In numerical mathematics, he has given an important contribution, a monograph An Analysis of Number Intervals. With this monograph, interval numerical analysis has received an stimulus. At the very beginning of the 20th century, in the era of analytic theory of functions, Petrović studied functions the Taylor series of which do not have zeros in appropriate circle of convergence. Renowned mathematicians Landau, Hardy Fejér, Montel, Pólya, were very interested and have studied the solutions Petrović had obtained in this field, while German mathematician Jentsch has elaborated these results further in his doctoral dissertation. The well-known Petrović’s inequality is the result associated with convex functions. It served as an inspiration to several of his pupils to study inequalities and to achieve significant success in this area. The textbook monograph Elliptic Functions is even today a favorite read in this important area of analysis. This does not exhaust the range of Petrović’s mathematical interests. For example, in several papers he engaged in determining – computing integrals with the use of series.
Petrović also produced work in the field of algebraic equations. In this area, particularly interesting is his work on geometry of zeros of a polynomial, which was also studied by the said group of famous mathematicians Landau, Hardy Fejér, Montel, Pólya. He determined a circle in which an algebraic equation has at least one root without using Rouché’s theorem. His paper from 1899, published in Comtes Rendus, is the first paper which determines the number of zeros contained in the given circle.
Reading academic papers by Mihailo Petrović in the period after the Great War, a gradual decline in quality is noticeable. Papers are less deep, but still bear certain ideas that have perhaps been already propounded, but which are nevertheless instilled with new energy. Contrary to contemporary style, Mihailo Petrović exhibits his results going from the specific towards the general. He had an ease of writing and in his manuscripts, as in a tale, he would first expound simple conclusions which he would gradually generalize and finally fully explain and transform into axioms. Such an approach has enabled a reader to easily follow his argument and understand the goals of the manuscript from its starting point.



Doctoral students of Professor Petrović: Tadija Pejović, Konstantin Orlov, Dragoljub Marković and Jovan Karamata (Faculty of Mathematics, University of Belgrade)
Among his academic achievements, the monograph Mathematical Specters (which is not related to contemporary, very developed, spectral theory of operators) in which Petrović, analogous to the light spectrums, develops a theory of mathematical specters. This very comprehensive, voluminous and original work by Petrović does not belong to analysis, but is closer to theory of numbers and cryptography, the disciplines he also showed an interest in. The basic idea of this theory of his is to code infinite series of data with infinite decimal developments of real numbers and then to translate mathematical operations with data into certain numerical or combinatory procedures with numbers – their codes. Petrović was particularly interested in repetitions in groups of numbers and he has seen an analogy in that with physical spectrums. Even though he had written the book in French, had received praise by some French mathematicians, and held one semester of lectures at the Sorbonne, this theory nevertheless did not significantly take root. An interesting view on it is expressed by French mathematician Bul, who in his review of this work at one point says that “such analogies are risky and like arches of the shiniest rainbow can be very seductive, but also illusionary.” On the other hand, contemporary digital computers, as implied by their very name, do not do anything else but precisely employ elementary arithmetical and basic combinatory operations over binary codes of series of data, even though the semantics of our problem concerning data and the resolving algorithm is found at some utterly different place. Therefore we can consider this work by Petrović as simply a work that appeared ahead of its time. His student, professor Konstantin Orlov, one of the most prominent heirs of professor Petrović in the field of differential equations and numerical analysis, has dedicated some attention to this area in his dissertation.
The contributions which pertain to application of differential equations, given in his famous work Phenomenology, as well as in a series of papers describing models in physics and chemistry, are portrayed in separate texts in this Catalogue.

Mihailo Petrović Alas in his late years (SASA Archive, 14188/21)
Mihailo Petrović belonged to the generation of the greatest mathematicians in the fields of analytical theory of differential equations, which was then at the pinnacle of development and in that context one should perceive an extremely high academic level his research was managing to attain. Most of his doctoral students in their dissertations dealt with topics associated with qualitative analysis of certain classes of equations. However, there are not too many of their works which in the theoretical sense had gone further than what Mihailo Petrović had already done. In the late 19th and early 20th century, unlike the methods of classical theory of equations, new abstract theories based on essentially new approaches in the analysis in the creation of mathematical models began to appear. The lineage of Mihailo Petrović, and subsequently his pupils in the fields of differential equations, seems to have followed less the development of mathematical physics or other branches of mathematics in which results of Painlevé and Picard have become dominant. The theory of partial differential equations has been insufficiently followed, which developed very quickly both in theoretical sense and in the sense of applications in almost all natural and technical sciences. Even Petrović himself did not follow new lines of development of theory of partial differential equations with altogether new methods stemming from areas topical at the time, namely set-theoretic topology and algebraic topology, geometry and algebra and in particular functional analysis. The results of Hilbert, Lebesgue, Dirac, Banach, Sobolev and many others have brought about completely new perspectives on mathematical investigations and their connection with other fields of science. This advance and development of mathematics globally was particularly intensive after the First World War. Certainly one should take into account that Mihailo Petrović was already fifty at the time, and that for a long time he remained outside the scientific mainstream, and could not stay abreast of the currents in science at the time due to complicated circumstances in Serbia after the Great War.
Mihailo Petrović was rather a ‘loner’ as a scholar. All papers, except one together with Karamata, he authored alone. The sole collaboration is interesting because it corrects one mistake made by Poincaré. The reason for this is his extreme individuality. He did not always elaborate ideas to the end, so that others, taking into account his results and ideas, have written more profound papers which, among other things, have also been more often quoted. Among colleagues, there were none who particularly promoted him also due to the fact that all of them, and here I refer to mathematicians in France, primarily sought after and were primarily aspiring to personal prestige, despite Petrović’s generous nature and propensity to form genial friendships. Given that at the time there were few mathematicians in Serbia and that Petrović was our first mathematician who addressed these problems, he could not experience significant mathematical support in Serbia, especially in the period between the two World Wars.

Certificate of membership in the Czechoslovakian Mathematical Society (SASA Archive, 14188/31)
Mihailo Petrović was not very interested in being referenced by other authors and it did not interest him whether other mathematicians read his work or not. On the other hand, mathematicians at the end of the 19th and the beginning of the 20th century did not have many possibilities for quoting other authors, and did not have the habit of such mutual academic behavior. In the dissertation of Mihailo Petrović, as well as in his other papers, quoting of results of other mathematicians does not appear in the form which is generally established today. In the field in which Petrović worked, citiations most often referred to results of Picard, Fuchs and certainly unparalleled, Painlevé. Mihailo Petrović himself in his dissertation has quoted very few sources, giving only eight references. The papers by Mihailo Petrović were quoted at the time close to the period in which he wrote them, but we think it was too insufficient. The papers in Acta Mathematica, Mathematical Annals (Matematički anali) and a series of other journals, dedicated to the problematics addressed in his doctoral dissertation, as well as most other papers, were quoted in late 19th and early 20th century. The results of Petrović’s first paper published in Comtes Rendus are cited in full in the famous and most highly acclaimed monographic book by Picard at the time, while the results of the doctoral dissertation are cited in Encyclopedia of Mathematics. However, in the vortex of events of the Balkan wars and the First World War, Mihailo Petrović slowly became less and less present in France, which also meant in world circles in which new science was created and developed.
Mihailo Petrović presented his work at a series of important international congresses of mathematics in Paris, Rome, Cambridge, Toronto and a conference of academic federations of France (a dozen times), Romania, Italy, Slavic countries and Balkan countries.
Petrović was appreciated and valued-esteemed as a scientist not only in Serbia but also in all of Europe. He was a member of Yugoslav Academy of Sciences and Arts, Czech Royal Academy, Polish Academy of Sciences in Krakow, Academy of Sciences in Warsaw, Romanian Academy of Sciences and a whole series of mathematical societies in Paris, Palermo, Bucharest, Leipzig, Prague, Lviv and in Paris he was a member of several scientific societies and academic associations.
His contribution in the area of teaching and education
Acting in the role he assumed due to fortunate circumstance, academician Petrović was of first-rate importance for the development of university teaching of mathematics in Serbia. In the period up to the First World War, as well as in the inter-war period, Professor Petrović was developing, almost alone, the university system of mathematical education of Serbia. He was a full professor of mathematics since 1894 at the Grand School at the Faculty of Philosophy and since 1905 at the University of Belgrade. He was the only one who supervised mathematical PhDs at the University of Belgrade from 1912 to 1941. He did not publish a lot of textbooks, only three, but his hand-written manuscripts are of extraordinary quality and a genuine pleasure to read even today. He held as much as 16 different courses: 10 courses in analysis and differential equations, 2 courses in algebra, 3 courses in numerical mathematics and a special course in phenomenology. He wrote eight manuscripts. He was a member of the commission for taking the professorship exam, was an envoy of the Ministry for the maturity exams, the president of the Supreme Educational Council of Serbia, a reviewer of high-school textbooks and published several papers dedicated to teaching methods.



Mathematical offspring of Professor Petrović: academician Slobodan Aljančić (Archive of the Mathematical Institute of SASA (MISASA)), academician Bogoljub Stanković (author: Dragan Aćimović, 2016) and professor Slaviša Prešić (author: Dragi Radojević, 2006)
Mihailo Petrović was a strict and principled professor. It has been noted that he held not a single public speech, although this is not unusual for a mathematician. With Milutin Milanković and Anton Bilimović in 1932, he established a journal entitled Publications de l’Institut Mathématique. The results of a greater engagement in his teaching work remains unknown for many of our mathematicians, who are mathematical heirs of Professor Petrović. A great number of mathematicians in Serbia have Mihailo Petrović Alas as their mathematical predecessor. In addition to students of academician Đura Kurepa and Serbian mathematicians who graduated abroad under the supervision of various professors, as well as geometricians whose predecessor was Danilo Blanuša, a great number among us have Mihailo Petrović as their close mathematical ancestor. Let us enumerate the names of his students of the first generation: professors Miroslav Berić, Sima Marković, Dragoljub Mitrinović, Konstantin Orlov, Tadija Pejović, Danilo Mihnjević, Miloš Radojčić. Among the first generation are also academicians Radivoj Kašanin and Jovan Karamata, and academicians among the second generation are Vojislav Avakumović, Miodrag Tomić, Slobodan Aljančić, Vojislav Marić, Milosav Marjanović, Dragoš Cvetković, Gradimir Milovanović, and among the third generation members of the Academy include Ivan Gutman, the correspondent member Miodrag Mateljević and the author of this article.
I feel greatly obliged to acknowledge our very renowned deceased mathamaticians who are descendents of academician Petrović, namely professors Manojlo Marović, Ernest Stipanić, Tatomir Anđelić, Milorad Bertolino, Milica Dajović and Vojin Dajović, Petar Vasić, Slaviša Prešič, Milivoj Lazić, Zagorka Šnajder, Svetozar Milić, Zoran Ivković, Janez Ušan, Dušan Adamović, Dragoljub Aranđelović, Vladeta Vučković, Bogdan Bajšanski, Ranko Vojanić, Tatjana Ostrogorski, Zoran Popstojanović, Ljuba Protić, Rade Dacić… All of them are have had a connection with academician Mihailo Petrović. Many names have not been mentioned. The information about them, as well as about those who are still active, can be found in the mathematical genealogy of academician Petrović, which is a part of this Catalogue.
His work in the Academy of Sciences and Arts

Academicians Mihailo Petrović, Bogdan Gavrilović and Milutin Milanković (Faculty of Mathematics, University of Belgrade)
As has already been stated, Mihailo Petrović Alas became a correspondent member of the Serbian Royal Academy in 1897 and a full member in 1899. The ceremonial reception was given in 1900, the same year when Jovan Cvijić became a full member of the Serbian Royal Academy. Academician Petrović engaged very actively in Academy’s work. He was a secretary of the Department of Natural Sciences as well as a member of the Executive Board of the Academy. He wrote reviews of mathematical papers published in Glas Academije (Academy Gazette) and presented new candidatess for membership in the Academy. In Glas Srpske kraljevske akademije (Serbian Royal Academy Gazette) he published some sixty papers, thus significantly raising the reputation of the Academy. In accordance with the tradition we still pursue and honor today, and which has been assumed from the tradition of the French Academy, works by authors outside the Academy were referred to by Academy members. In these activities, Mihailo Petrović was very active, particularly due to the fact that his students published a great number of their most important papers precisely in Glas Srpske kraljevske akademije. He promoted an entire series of academicians, for example Bogdan Gavrilović in 1900, Milutin Milanković, Anton Bilinović and Jovan Karamata.
Jovan Cvijić, Mihailo Petrović and Bogdan Gavrilović invited in 1909 Milutin Milanković to transfer from Vienna to the University of Belgrade, which he accepted. They proposed Milanković for correspondent member of the Academy in 1920 and for full member in 1925. When Jovan Cvijić died in 1927, Petrović was the most serious candidate for the president of the Serbian Royal Academy, however Slobodan Jovanović was eventually elected.

The Academy building, constructed in 1924, designed by Dragutin Đorđević and Andre Stevanović in the style of academic realism, with elements of art nouveau (author: Dragan Aćimović, 2018)
Jovan Karamata was promoted to an Academy member in 1939. To his teacher, Mihailo Petrović, he provided great assistance in systematizing academic publications. It is owing to him and to the great desire of Mihailo Petrović to catalogue his papers and his legacy that today we have a comparitive wealth of data about his life and work.